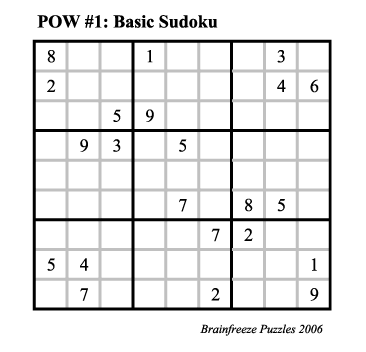
We'll start our Special All-Sudoku Semester with a basic run-of-the-mill Sudoku puzzle. The rules are simple: You must fill in the 9x9 grid in such a way that each row, each column, and each of the nine marked 3x3 blocks contain all of the integers from 1 through 9 exactly once. Some initial conditions are given, and the usual convention is for these initial conditions to display 180 degree rotational symmetry. Sudoku puzzles are always constructed to have a unique solution. The puzzle below is of medium-hard difficulty. If you're new to Sudoku, you might want to start by practicing some easier puzzles; the website www.websudoku.com has lots of puzzles of varying difficulty.

Sudoku puzzles are not only fun; they are also the subject of mathematical
research. For example, it has been shown that there are
6,670,903,752,021,072,936,960 possible
completed Sudoku grids (Felgenhauer and Jarvis; see
www.afjarvis.staff.shef.ac.uk/sudoku/). It has been conjectured, but
not proven, that 17 is the minimal number of
initial conditions that are required
for a Sudoku puzzle to have a unique solution. Over 35,000 puzzles with 17
initial conditons have been found, but no puzzles with 16 initial conditions
have yet been found (see www.csse.uwa.edu.au/~gordon/sudokumin.php). It has also been
conjectured that if the initial conditions are to have the traditional
rotational symmetry, that 18 initial conditions are needed. Obviously,
fewer intitial conditions can lead to a more challenging puzzle.
For comparison,
consider that this week's puzzle has 22 initial conditions.
Rules, solutions, and winners listed at
www.math.jmu.edu/~taal/POW.
Solutions are due by noon on Tuesday, January 17, 2006.
Return solutions ON PAPER to Laura Taalman, Burruss 127, MSC 7803.
Include your name and email address with your solution.